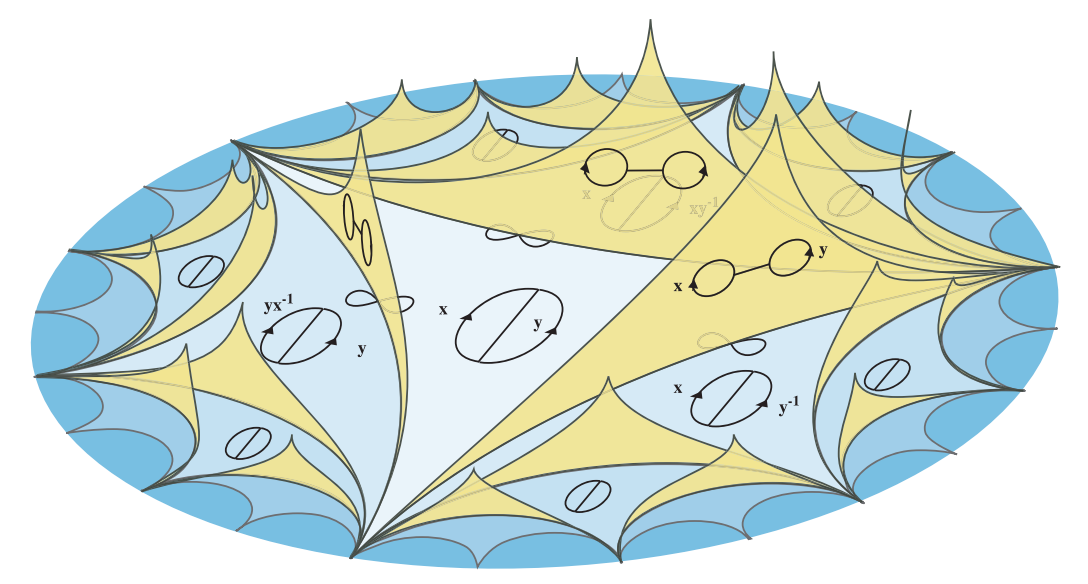
(A picture of the outer space for $F_2$, shamelessly borrowed from Karen Vogtmann)

Research Interests

For the expert

Geometric group theory is a field which aims to study infinite, finitely generated groups as geometric objects using tools from geometry, topology, and dynamics. Much of my past and present research has focused on outer automorphisms of free groups. Specifically, the extent to which they can be understood through analogies with mapping class groups of finite type surfaces. Current, past, and future projects can be found below, on my CV, and on my research statement.

In a rather different direction, I've recently been learning some commutative algebra & algebraic geometry. In work with Adam Boocher, we used Boij-Soederberg theory to find strong lower bounds for the Betti numbers of modules over a polynomial ring that have small regularity relative to the minimal degree of a first syzygy.

Electronic copies of my papers are linked below.

Here is my academic curriculum vitae.

For the novice

My research lies at the intersection of geometry and algebra. A geometric group theorist aims to understand algebraic objects, called groups, via their interactions with geometric objects (spaces). My favorite group is called $Out(F_n)$: the outer automorphism group of the free group $F_n$. To learn about $Out(F_n)$, we need one (or many) spaces that this group interacts with. There is a close relationship between $F_n$ and graphs (see picture), which gives rise to an action of $Out(F_n)$ on the space of all such graphs...outer space. By studying/understanding outer space (not the one up in the sky) and the way that $Out(F_n)$ acts on it, we can learn about the group I like so much. Much of the current understanding of $Out(F_n)$ comes from drawing analogies with better understood groups like the mapping class group, or the modular group. There are also close ties between these groups and hyperbolic geometry.

[5]. Farrell-Jones Conjecture for hyperbolic-by-cyclic groups (with M. Bestvina, K. Fujiwara) submitted to International Mathematics Research Notices
[4]. Large lower bounds for the Betti numbers of graded modules with low regularity (with A. Boocher) published in International Journal of Algebra and Computation
[3]. Farrell-Jones Conjecture for free-by-cyclic groups (with M. Bestvina, K. Fujiwara)
[2]. Loxodromics for the cyclic splitting complex (with R. Gupta) published in Pacific Journal of Mathematics
[1]. Distortion for abelian subgroups of Out(Fn) published in International Journal of Algebra & Computation